Mesoscopic numerical simulation of the mechanical properties of concrete creep

Song, Chen; Jun, Lu

Journal of Vibroengineering

Browse Journal

Submit article

Published: 31 December 2013

Check for updates

Mesoscopic numerical simulation of the mechanical properties of concrete creep

Chen Song¹

Lu Jun²

¹Reservoir Dams Safety Monitoring Centre of Chongqing, Chongqing, 401147, P. R. China

²Nanjing Hydraulic Research Institute, 34 Hujuguan Road, 210029, Nanjing, Jiangsu, China

Corresponding Author:

Lu Jun

Cite the article Download PDF

Downloads 1243

Abstract

In order to carry out creep mechanism research on concrete under mesoscopic scales, Monte Carlo method has been used to put forward the meshing method of aggregate and mortar under mesoscopic scales, while combining the theory of mixture and the porous medium theory to establish the composite material model of aggregate, cement mortar and the moisture content under mesoscopic scales. Concrete aggregate random distribution and meshing program and concrete creep simulation analysis program under meso-scale have been prepared. The simulation analysis shows that the program can effectively simulate law of creep motion inside the concrete.

1. Introduction

Concrete materials is a kind of typical porous medium, which contains aggregate and cement paste and a certain amount of moisture, the concrete under the action of forces will generate creep deformation as time grows, there are a variety of theories currently to explain the mechanism of concrete creep, generally put forward in the basis of the mesoscopic structure of cement paste, mainly including viscoelastic theory, seepage theory, viscous flow theory, the plastic flow theory, the internal force equilibrium theory and the theory of micro cracks, etc. [1], but so far, none of which have been widely accepted. The universal opinion on the concrete moisture nowadays is: the existence of vaporizable free water inside the concrete is primary for creep [1]. Therefore the coupling effect of cement mortar, free water and aggregate needs to be considered during the process that the concrete creep model is established [2, 3], the relevant scholars have combined with the theory of porous media to present creep finite element model which is capable to well explain effect of the moisture existence on the macro creep [4], but fails to show micro-analysis of the changes inside the concrete.

In this paper, on the basis of related research [5-6], the relevant theories and mathematical methods are used to attempt explaining concrete creep mechanism from meso-scale. The innovation points of this paper: 1) use Monte Carlo method, etc. to randomly generate discrete particles meeting certain distribution, generate composite material model consisting of aggregate, cement mortar; 2) use the theory of mixture to respectively homogenize aggregate and mortar, combine the theory of porous media to introduce water factor into aggregate and mortar, establish the composite material model of aggregate, cement mortar and moisture content; (3) in accordance with the relevant theory, the concrete aggregate random distribution and meshing program, concrete creep under simulation analysis program under meso-scale.

2. Aggregate randomly generated

2.1. Concrete aggregate gradation

Aggregate of concrete is divided into fine aggregate and coarse aggregate [7], the size of the aggregate can be indicated by particle distribution curve (or integral curve) which has limited that the size should not be greater than the percentage of a given particle as a function of average particle size. In the beginning of the 20th century, W. B. Fuller and other scholars in the United States [8], relying on screening test results from a lot of testing work, have put forward the ideal distribution curve of maximum density. The basis for Fuller grading theory is to arrange and match regularly aggregate particles of concrete material according to size and thickness to become mixture with maximum density and minimum gap. Fuller's ideal gradation curve indicates grain composition as well as parabola below the fine aggregate, its equation is:

1

P = 100 \sqrt{\frac{D}{D_{max}}},

of which: $P$ represents mass percent of the aggregate passing sieve pore $D$ ; $D$ represents the sieve diameter; $D_{max}$ is the maximum particle size.

The concrete in this design has high strength, good permeability, saving cement. For simplicity, the aggregate particle of aggregates such as pebble and gravel in shape of ball or round is presumed to be spherical, Fuller curve is used to determine the three-dimensional size distribution curve of the aggregate, the concrete poured by such grading can generate optimized structure density and strength [9], which can solve the problem of concrete aggregate gradation and random distribution in combination with Monte Carlo method [10].

2.2. Aggregate random distribution

By using Monte Carlo method, assume $x$ as random variables in uniform distribution on interval of [0, 1], the computer can help to work out sampling sequence ${x_{n}}$ of random variable $x$ , usually $x_{n}$ is known as random number of random variable $x$ in uniform distribution on interval of [0, 1].

Assume that the coordinate of the concrete block is $x \in [x_{m i n}, x_{m a x}]$ , $y \in [y_{m i n}, y_{m a x}]$ , $z \in [z_{m i n}, z_{m a x}]$ , if the central point of aggregates $(x_{i}^{c}, y_{i}^{c}, z_{i}^{c})$ is in uniform distribution in the concrete block, then:

2

\{\begin{array}{l} x_{i}^{c} = x_{m i n} + (x_{m a x} - x_{m i n}) x_{i}, \\ y_{i}^{c} = y_{m i n} + (y_{m a x} - y_{m i n}) y_{i}, \\ z_{i}^{c} = z_{m i n} + (z_{m a x} - z_{m i n}) z_{i}, \end{array} (i = 1, n) .

For the problem of two-dimensions, the cumulative distribution function can be used to represent probability that any point with a diameter on one inner section inside the concrete [9]:

3

P_{c} (D < D_{0}) = P_{k} \times [\begin{matrix} 1.065 {(D_{0} / D_{m a x})}^{1 / 2} - 0.053 {(D_{0} / D_{m a x})}^{4} - 0.012 {(D_{0} / D_{m a x})}^{6} - \\ 0.0045 {(D_{0} / D_{m a x})}^{8} + 0.0025 {(D_{0} / D_{m a x})}^{10} \end{matrix}],

of which: $P_{k}$ is the percentage of the aggregate volume and the total volume of concrete; $D_{m a x}$ is maximum particle size of the aggregate.

According to the Eq. (3), aggregate distribution inside the concrete can be generated.

The above method can be used to produce composite material concrete specimen model of random aggregate and mortar, realize mesh generation of aggregate and mortar under the mesoscopic scales, record unit and node information of aggregate and mortar, combine with the mixture theory and theory of porous media, calculation model of water concrete aggregate and mortar can be set up.

3. Concrete creep model based on the theory of the porous medium

It is generally acknowledged that concrete aggregate is inert, whose instantaneous elastic deformation only under outer loads is extremely small, generally 3~5×10^-6/MPa, so it can approximately be considered that aggregate creep deformation doesn’t occur but only generates elastic deformation [1], the slurry has obvious creep properties, therefore different mechanical properties of aggregate and mortar inside the concrete should be considered.

When establishment of calculation model, besides considering different mechanical properties of aggregate and mortar, problems still need to be considered: (1) the water as the important factors influencing the creep needs to establish quality and movement balance equation of the moisture migration movement of the aggregate and mortar; (2) aggregate and mortar as two kinds of different materials, have different moisture contents, whose moisture contents need to be considered separately; (3) coupling calculation model of aggregate, mortar and water should be easily calculated.

In order to solve the above problem, this paper combines with mixture theory [12], penalty parameter method, Galerkin weighted residual method [13] and implicit expression solution for creep stress analysis proposed by Zhu Bofang[14], concrete is considered as continuous homogeneous medium consisting of solid phase (aggregate and mortar) and moisture, two phases of solid and liquid evenly and continuously disperse in control space regardless of the specific distribution characteristics of concrete solid phase skeleton and geometric features of pore structure, uses the frictional resistance flowing between two phases of micro solid and liquid to realize fluid-structure interaction, micro solid skeleton and water are incompressible, and by ignoring the physical strength and pore fluid viscosity, basing on mixture theory, it can be concluded that controlling field equation of concrete porous medium model:

4

\nabla \cdot (φ^{S} {\dot{u}}^{S} + φ^{F} {\dot{u}}^{F}) = 0,

5

ρ^{S} {\ddot{u}}^{S} = \nabla \cdot T_{e}^{S} - φ^{S} \nabla p + α ({\dot{u}}^{F} - {\dot{u}}^{S}),

6

ρ^{F} {\ddot{u}}^{F} = - φ^{F} \nabla p - α ({\dot{u}}^{F} - {\dot{u}}^{S}),

where: $\cdot$ represents conclusion of material derivative for time, corner mark $α$ represents solid skeleton $(α = S)$ and pore fluid $(α = F)$ , $\nabla$ are Nabla operators, $u^{α}$ is displacement of group $α$ ; $ρ^{α}$ is macro density ( $ρ^{α} = φ^{α} ρ^{α R}$ , $ρ^{α R}$ is the true density of corresponding components); $φ^{α}$ is volume fraction (meet the saturation constraints $φ^{S} + φ^{F} = 1$ ), $p$ is the pore pressure, $T_{e}^{S}$ is effective stress tensor of the solid skeleton, $α_{v}$ is diffusion resistance coefficient, for isotropic continuum:

7

α_{v} = {(φ^{F})}^{2} ρ^{F} g / k^{F},

of which: $k^{F}$ is Darcy permeability coefficient; $g$ is acceleration of gravity.

Aggregate skeleton constitutive equation is:

8

T_{e}^{S} = 2 μ^{S} E^{S} + λ^{S} (E^{S} \cdot I) I .

Among them:

9

E^{S} = \frac{1}{2} (\nabla u^{S} + \nabla^{T} u^{S}) .

Mortar skeletons are considered as linear elastic creep, and according to creep implicit expression method derived by Zhu Bofang, concrete strain increment consists of the three parts of elastic strain increment and creep strain increment, temperature strain increment:

of which: $Δ ε_{n}$ is strain increment array; $Δ ε_{n}^{e}$ is the elastic strain increment array; $Δ ε_{n}^{c}$ is creep strain increment array; $Δ ε_{n}^{I}$ is the temperature strain increment array.

Eliminate air compressor by using penalty parameter method, Galerkin weighted residual method can be based on to respectively deduce finite element basic equation of two phases of solid and liquid of aggregate and moisture:

10

[\begin{matrix} M_{n}^{S} & 0 \\ 0 & M_{n}^{F} \end{matrix}] \{\begin{matrix} {\ddot{u}}_{n}^{S} \\ {\ddot{u}}_{n}^{F} \end{matrix}\} + (β [\begin{matrix} {(φ^{S})}^{2} C_{n} & φ^{S} φ^{F} C_{n} \\ φ^{S} φ^{F} C_{n} & {(φ^{F})}^{2} C_{n} \end{matrix}] + [\begin{matrix} A_{n} & - A_{n} \\ - A_{n} & A_{n} \end{matrix}]) \{\begin{matrix} {\dot{u}}_{n}^{S} \\ {\dot{u}}_{n}^{F} \end{matrix}\} + [\begin{matrix} K_{n} & 0 \\ 0 & 0 \end{matrix}] \{\begin{matrix} u_{n}^{S} \\ u_{n}^{F} \end{matrix}\} = \{\begin{matrix} R_{n}^{S} \\ R_{n}^{F} \end{matrix}\} .

Among them:

11

M_{n}^{S} = \int_{v_{n}} ρ^{S} N^{Τ} N d v, M_{n}^{F} = \int_{v_{n}} ρ^{F} N^{Τ} N d v, A_{n} = \int_{v_{n}} α_{v} N^{Τ} N d v,

C_{n} = \int_{v_{n}} B^{Τ} D_{1} B d v, R_{n}^{F} = \int_{Γ_{t^{F}}} N^{Τ} {\hat{t}}^{F} d Γ .

(1) Two-phase finite element basic equation of aggregate and water:

12

K_{n} = \int_{v_{n}} B^{Τ} {\bar{D}}^{S} B d v, R_{n}^{S} = \int_{Γ_{t^{s}}} N^{Τ} {\hat{t}}^{S} d Γ .

Aggregate elastic stress-strain relationship matrix $\bar{D}$ is expressed as:

13

\bar{D} = \frac{E (1 - ν)}{(1 + ν) (1 - 2 ν)} [\begin{matrix} 1 & \frac{ν}{1 - ν} & \frac{ν}{1 - ν} & 0 & 0 & 0 \\ 1 & \frac{ν}{1 - ν} & 0 & 0 & 0 \\ 1 & 0 & 0 & 0 \\ \frac{1 - 2 ν}{2 (1 - ν)} & 0 & 0 \\ \frac{1 - 2 ν}{2 (1 - ν)} & 0 \\ \frac{1 - 2 ν}{2 (1 - ν)} \end{matrix}] .

(2) In two-phase finite element basic equations of slurry and water:

14

K_{n} = \int_{v_{n}} B^{Τ} {\bar{D}}_{n} B d v, R_{n}^{S} = \int_{v_{n}} B^{Τ} {\bar{D}}_{n} η_{n} d v + \int_{Γ_{t^{s}}} N^{Τ} {\hat{t}}^{S} d Γ .

Mortar creep stress strain relations matrix expression ${\bar{D}}_{n}$ is expressed as:

15

{\bar{D}}_{n} = \frac{E (t_{n - 0.5})}{1 + q_{n} E (t_{n - 0.5})} Α^{- 1},

16

q_{n} = C (t_{n}, t_{n - 0.5}),

17

η_{n} = \sum_{j = 1}^{m} (1 - e^{- r_{j} Δ t_{n}}) ω_{j n},

18

ω_{j n} = ω_{j, n - 1} e^{- r_{j} Δ t_{n - 1}} + Α Δ σ φ_{j} (t_{n - 1 - 0.5}) e^{- 0.5 r_{j} Δ t_{n - 1}},

19

ω_{j 1} = Α Δ σ_{0} φ_{j} (t_{0}),

20

C (t, τ) = φ (τ) [1 - e^{- r (t - τ)}],

of which: $C (t, τ)$ is creep function; $t$ is loading time; $τ$ is the loading age.

Sum over all units of the whole area, the system equations can be concluded:

21

M \ddot{u} + J \dot{u} + p = R,

of which: $M$ , $J$ , 𝐩, $R$ , is respectively, the corresponding unit matrix and vector set.

4. Numerical simulation and analysis of uniaxial compression creep characteristics

According to the above theory and formula derivation, the concrete aggregate random distribution and mesh generation program has been compiled, concrete creep simulation analysis program under meso-scale, in order to verify the reliability of the program, for the concrete specimen shown in Fig. 1 under uniaxial compression creep finite element analysis, in order to simplify the calculation, it is considered as the plane strain problem. Aggregate maximum diameter $D_{m a x}$ is 0.006 m, the percentage of aggregate volume and the total volume of concrete is 19 %. Its top uniformly suffers from compressive stress function with distributed load of 12 MPa to constraint the displacement to $x$ and $y$ directions at the bottom. Through experiment [1] and related reference literature [5], specific calculation material parameters have been worked out and shown in Table 1. The expression of calculation method for cement mortar creep as well as the creep degree of elastic modulus according to reference [10] are:

$E = 2.625 (1 - e^{- 0.4 τ^{0.34}}) \times 10^{4} MPa$ ,

$C (t, τ) = (8.8 \times 10^{- 6} + 8.1 \times 10^{- 5} τ^{- 0.45}) (1 - e^{- 0.3 (t - τ)})$

$+ (1.98 \times 10^{- 5} + 3.37 \times 10^{- 5} τ^{- 0.45}) (1 - e^{- 0.005 (t - τ)}) 1 / MPa$ .

Table 1Material parameters of concrete

Item	Density of the solid phase $ρ^{S}$ (kg/m³)	Liquid phase density $ρ^{F}$ (kg/m³)	Modulus of elasticity $E$ (MPa)	Poisson's ratio $ν$	Liquid phase volume fraction $n$ (%)	Coefficient of permeability $k$ (m/s)
Aggregate	2700.0	1000.0	70000.0	0.167	0.05	3.65×10^-4
Cement mortar	2000.0	1000.0	25000.0	0.200	5.00	3.65×10^-2

Fig. 1Concrete calculation models

Direction of the arrows in Fig. 2 represents the flow direction and the length of the arrow represents the size of the flow velocity. Fig. 3 shows mesh deformation picture within 90 days. The internal space liquid of concrete surface settlement caused by the action of forces drains out. The permeability coefficient of internal aggregate liquidity is poorer and its flow velocity is smaller. Mortar has larger permeability coefficient and better liquidity, but because the aggregate restricts its movement direction, the liquid flows in the mortar along the direction of edges tangent of aggregate.

Fig. 2Vector diagram for 90 days of gap liquid flow field

Fig. 3Diagram for 90 days of mesh deformation

Fig. 4 can show clearly the creep movement characteristics inside the concrete under the action of forces from the top. The lower concrete surface is regarded as the datum plane, whose displacement constrains the corresponding displacement of its nine nodes to become larger along the increase of the distance. Curve shows that creep displacement increase of concrete is fastest during the first 30 days, stable on the 300th day, and can be known never to increase on the 360th day.

Fig. 4Time history of the vertical solid phase displacement of concrete at different nodes

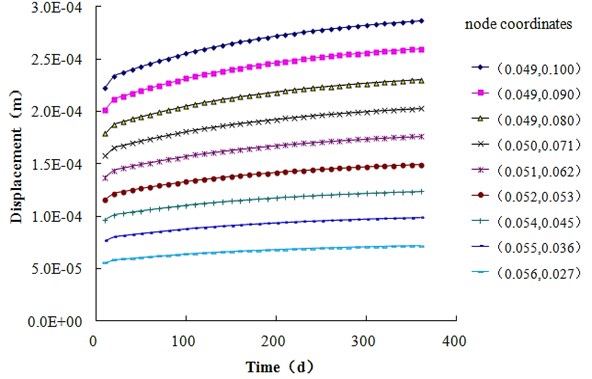
Table 2 shows relation between internal pore pressure and liquid motion inside the concrete, combined with Fig. 4, it indicates that when concrete creep displacement changed fast on the first 30 days, the liquid rate was larger; on the 180th day, liquid and solid movement slowed down, pore pressure also began to gradually dissipate; on the 360th day, pore pressure was very small, the corresponding displacement of solid phase and liquid phase velocity respectively reached a maximum value in their separate calculations, which shows that close relations of visible pore pressure, displacement of solid phase as well as liquid phase are consistent with actual micro movements.

Table 2Calculated value of the liquid phase and the pore pressure

Time (d)	3	7	28	90	180	300	360
Velocity of the liquid phase (mm/d)	1.09×10^-1	4.01×10^-2	1.99×10^-3	1.43×10^-3	9.35×10^-4	4.23×10^-4	3.14×10^-4
Pore pressure (Pa)	42400	20700	4780	2434	150	132	122

Fig. 5 changes of strain nephogram over time can show that under the action of forces at the top, strain of internal mortar and aggregate continues to increase. The energy conversion between mortar and aggregate due to the mortar in the process of flow hindered by aggregate, mortar strain around the aggregate is larger. Along time increase, the rise of elastic modulus and strength, the movement of the mortar slows down, and mortar strain also gradually homogenizes.

Fig. 5Figure of principal strain evolution over time

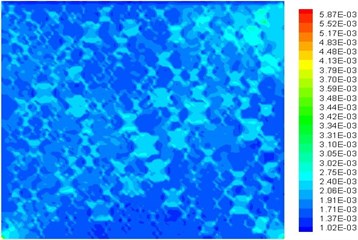
a) 10 days

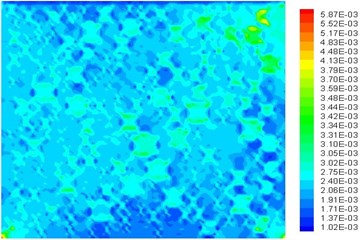
b) 180 days

Fig. 6 shows that when concrete suffers load, the water slurry early has the characteristics of liquidity and the elastic modulus increasing along with time, which enables the aggregate system to suffer higher stress in the initial stage, while, the water slurry suffers less stress at this time along with the time growth, due to increase of the mortar mechanical performance index, to the load increases leads to the occurrence and development of creep effect, continuous redistribution of internal stress of concrete mortar and aggregate, aggregate stress decreases gradually over time, and mortar stress increases slowly as a result of the action of aggregate.

Fig. 6For vertical stress evolution over time (MPa)

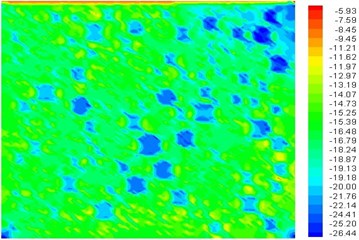
a) 10 days

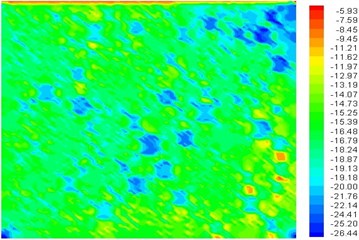
b) 180 days

5. Conclusions

This paper proposes a new method based on mesoscopic scales to research concrete creep process. By adopting the method of Monte Carlo, the establishment of methods to achieve mesh information of aggregate and cement mortar, concrete creep calculation model under the mesoscopic scales through the porous media theory, and introduction of water influence factors into concrete creep calculation model. The similarities and differences between the method in this paper and the existing creep theories: water movement is close to the seepage theory, in this paper, the moisture refers to free water, different from seepage theory to subdivide water into gelled water (absorbed water and interlayer water); on the assumption of concrete solid phase, it is relatively similar with viscous flow theory which divides concrete into cement paste in viscous flow and inert aggregate without flow, the solid phase of the model in this paper is divided into cement paste in creep deformation and aggregate in elastic deformation; in assumption on the mechanical properties of solid model, it is close to viscous elasticity theory which regards the cement paste as elastic gel skeleton and complex consisting of viscoelastic fluid filling the gap, this paper omits the viscosity of water for the model in order to simplify the calculation, and regards cement paste as cement gel skeleton in creep deformation and complex consisting of non-viscous water filling the gap. As described in the literature, it is more suitable to combine several theories to explain the creep mechanism. The numerical calculation shows that this paper can well describe the internal creep movement of concrete, and has certain significance in theory research.

References

Hui Rongyan, etc. The Creep of Concrete. China Railway Publishing House, Beijing, 1988.

Search CrossRef
Boer R. De. Theory of porous media: highlights in the historical development and current state. Springer Verlag, Berlin, Heidelberg, 2000, p. 634.

Search CrossRef
Schrefler B. A. Mechanics and thermodynamics of saturated/unsaturated porous materials and quantitative solution. Appl. Mech. Rev., Vol. 55, 2002, p. 351-388.

Search CrossRef
Chen Song, Ke Minyong, Hong Xiaolin. Finite element analysis based on the theory of porous media theory for mechanical behavior of concrete creep. Chinese Journal of Solid Mechanics, Vol. 30, Issue 5, 2009, p. 522-526.

Search CrossRef
Chen Song. Numerical Analysis of the Concrete Creep Process and Experimental Study. Doctoral Dissertation of Nanjing Hydraulic Research Institute, Nanjing, 2010.

Search CrossRef
Chen Song. The Dynamic Responses of Elastic Viscoplastic Porous Media under Different Yield Criterion. Master Dissertation of Chongqing University, Chongqing, 2007.

Search CrossRef
Mainly Edited of East China Institute of Water Conservancy. Hydraulic Design Handbook: 2 Geological Hydrological Construction Materials. Water Resources and Electric Power Press, Ed. 2, 1984.

Search CrossRef
Edelman I., Wilmanski K. Asymptotic analysis of surface waves at vacuum/porous medium and liquid/porous medium interfaces. Continuum Mech. Thermody., Vol. 14, 2002, p. 25-44.

Search CrossRef
Ju Hongchang. Mechanical Property Research Based on Mesoscopic Calculation Mechanics. College of Civil Engineering, Nanjing, HHU, 2008.

Search CrossRef
Chen Qiu, Liu Xianbin. Stochastic Finite Element Method and Its Engineering Application. SWJTU, Chengdu, 1993.

Search CrossRef
Owen D. R. J., Hinton E. Finite Elements in Plasticity: Theory and Practice. Pineridge Press Limited, Swansea, UK, 1980, p. 431-465.

Search CrossRef
Bowen R. M. Compressible porous media by use of the theory of mixtures. International Journal of Engineering Science, Vol. 20, 1982, p. 19-45.

Search CrossRef
Wang Xuchen. Basic Principle of Finite Element Method and Numerical Method. Tsinghua University Press, Beijing, 1997, p. 7-22.

Search CrossRef
Zhu Bofang. Principle and Application of FEM. China Water Power Press, Beijing, 2000, p. 345-360.

Search CrossRef

About this article

Received

13 August 2013

Accepted

02 September 2013

Published

31 December 2013

Keywords

reinforced concrete

creep

mesoscopic scales

Acknowledgements

This work is supported by the National High Technology Research and Development Program of China (863 Program, No. 2007AA11Z106), Natural Science Foundation Project of CQ CSTC (cstc2012jjA00025), the National Natural Science Foundation of China (51309163), and the Central Public Research Institutes for Basic Research Funds Project (Y413003). Thanks for the help.

This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.